High Order Phase Field Models
Functionalized polymer membranes are the work horses of modern green energy. These membranes act as Maxwell's Demons, letting ions flow through the membrane while forcing electrons to flow through an external path to complete the circuit. Such membranes are used in solar cells, batteries and fuel cells. Further, the way in which these membranes function is deeply related to the behavior of lipid bilayers in cells of living tissue.
At its simplest level, one has a conducting polymer that is soft that can do work. By itself, this conducting polymer is not useful because it is too soft. To overcome this issue, a rigid polymer is introduced to the system that can form a scaffolding. The scaffolding provides the skeleton why the conducting polymer does the desired work. One way of assembling such a system is to cast a membrane. When cast, one functionalizes the two polymers, that is a chain is added to one of the polymers that will make it hydrophobic and a different chain is added two the other polymer that makes if hydrophilic. This means that if the polymers were mixed, they would phase separate from one another. The polymers are mixed with a solvent that facilitates both the hydrophobic and hydrophilic polymer chains being intermingled. Then by drawing off the solvent, the polymers start to set and phase separate. Depending on many parameters in the system, including how the solvent is removed, one can change the morphology of the cast polymer.
Morphology of a membrane can be modeled accurately using detailed particle models, but these models can only simulate a few hundred picoseconds of the casting process. Typically, time associated with casting of these membranes is on may range from hours to days. Because of the lack of computationally tractable models that can be used to optimize the membrane casting in a virtual laboratory, the current approach to developing such membranes is Edisonian. This means it is an experimental process governed by trial and error. The introduction of a class of models that can simulate the cast structures of these membranes is of great use to this community.
This work centers on the idea that we can describe the evolution of polymer membranes with continuum models, known as phase field models. The most well-known phase field model is the Cahn-Hilliard Model for describing the evolution of grain boundaries in metals. Here we are working with a class of models known as the Functionalized Cahn-Hilliard Equations.
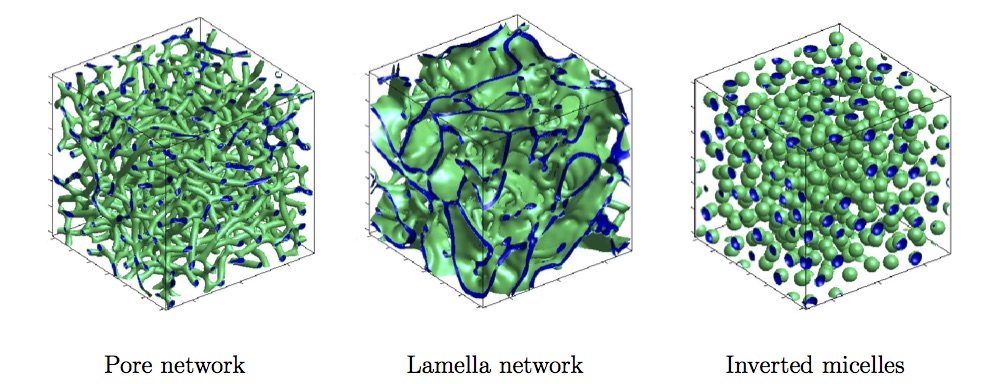
Above are the results of a new phase field model designed to capture the structures that are possible in these complex systems. By slight modifications of the chemical potential in this model, the model can dramatically change the types of structure that is predicted. These three morphologies are common in cast membranes and having a tool that can predict what type of structure is possible is key. Once we have the structure, modeling the transport properties of the membrane is a well understood process and one can seek to optimize the casting process to give the best properties possible.
This work is in collaboration with Professor Keith Promislow's group. Our efforts here center on the numerical challenges introduced by these models. While it is possible to simulate long time scales with these continuum models, they are particularly challenging numerically, having up to 6th order derivatives and 4th order non-linear terms.
Our most recent work has been to extend the method of lines transpose ideas to the area of phase field models to generate an \(O(N)\) implicit method that is gradient stable for both scalar and vector phase field models. We are heavily leveraging GPGPUs in our work on phase field models.
Select Papers:
- N. Gavish, J. Jones, Z. Xu, A.J. Christlieb and K. Promislow, "Variational Models of Network Formation and Ion Transport: Applications to Perfluorosulfonate Ionomer Membranes'', Polymers, 4(1), 630--655, 2012
- A.J. Chirstlieb, K. Promislow and Z. Xu "On the unconditionally gradient stable scheme for Cahn-Hilliard equation and its implementation with Fourier method'', Communications in Mathematical Sciences, 11(2),345--360, 2012
- J. Jones, Z. Xu, A.J. Christlieb, and K. Promislow, "Using GPGPU to Enhance Simulation of the Functionalized Cahn-Hilliard Equation'', 2012 IEEE Symposium on Application Accelerators in High Performance Computing (SAAHPC), 153--156, 2012
- K. Promislow, J. Jones, Z. Xu, N. Gavish and A.J. Christlieb, "Variational Models of Pore Networks in Ionomer Membranes: The Role of Electrostatics'', ECS Transactions, 50(2), 161--173, 2013
- A.J. Christlieb, J. Jones, K. Promislow, B. Wetton, M. Willoughby, "High accuracy solutions to energy gradient flows from material science models'', Journal of Computational Physics, 257, Part A, 193--215, 2014
- M. Causley, H. Cho, A.J. Christlieb, "Method of lines transpose: Energy gradient flows using direct operator inversion for phase field models'', SIAM Journal on Scientific Computing, 39(5), B968--B992, 2017